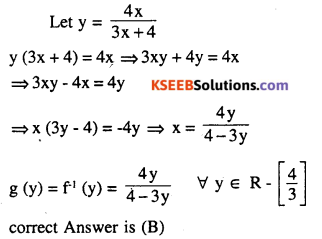2nd PUC Maths Question Bank Chapter 1 Relations and Functions Ex 1.3

Students can Download Maths Chapter 1 Relations and Functions Ex 1.3 Questions and Answers, Notes Pdf, 2nd PUC Maths Question Bank with Answers helps you to revise the complete Karnataka State Board Syllabus and score more marks in your examinations.

Karnataka 2nd PUC Maths Question Bank Chapter 1 Relations and Functions Ex 1.3

2nd PUC Maths Relations and Functions NCERT Text Book Questions and Answers Ex 1.3

Question 1.
Let f: {1, 3, 4} →  {1,2, 5} and g : {1, 2, 5} {1,3} be given by f= {(1,2), (3,5), (4,1)} and
g = {(1, 3), (2, 3), (5,1)}. Write down gof.
Answer:
gof (1) = g (f (1)) = g (2) = 3 gof (3)
= g {f (3)} = g (5) = 1
= gof (4) = g (f (4)) = g (1) = 3
∴ gof = {(1,3), (3,1), (4,3)}

Question 2.
Let f, g and h be functions from R to R. Show that
(f + g)oh =foh + goh
(f.g)oh = (foh) . (goh)
Answer:
∀ x ∈ R
{(f + g)oh} (x) = (f + g) h (x)
= f (h (x)) + g (h (x)) = foh (x) + goh (x)
= {foh + goh} (x)
∴ (f + g) oh = foh + goh
((fg )oh) (x) = (f.g) (h (x))
= fh (x). gh (x)
= {foh (x)} . {goh (x)}
{foh. goh} (x)
(fg) oh = (foh) (goh)

Question 3.
Find gof and fog, if
(i) f(x) = |x| and g(x) = |5x – 2|
Answer:

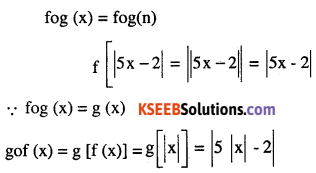
(ii) f (x) = 8x³ and g(x) = \(x^{\frac{1}{3}}\)
Answer:

Question 4.
If \(f(x)=\frac{(4 x+3)}{(6 x-4)}, x \neq \frac{2}{3}\),show that fof(x)= x,for all
\(x \neq \frac{2}{3}\),what is the inverse of f?
Answer:

Question 5.
State with reason whether following functions have inverse
(i) f : {1,2, 3, 4} → {10} with
f = {(1, 10), (2, 10), (3, 10), (4, 10)}

(ii) g : {5, 6, 7, 8} → {1, 2, 3, 4} with
g = {(5, 4), (6, 3), (7, 4), (8, 2)}

(iii) h : {2, 3, 4, 5} → {7, 9,11,13} with
h = {(2, 7), (3, 9), (4, 11), (5, 13)}
Answer:
(i) f (1) = f (2) = f (3) = f (4) = 10
⇒ f is not one-one
∴ f does not have an inverse

(ii) g(5) = 4  g(6) = 3 g(7) = 4 g(8) = 2 g(5) = g(7) = 4
∴ g is not one-one, hence g does not have inverse

(iii) h(2) = 7, h(3) = 9, h(4) = 11, h(5)=13,
∴  h is one-one

Also range of h = {7,9,11,13} = co-domain
⇒ h is onto
Hence h has an inverse
h^-1 = {(7,2), (9,3), (11,4), (13, 5)}.

Question 6.
Show that f: [-1,1] → R, given by f(x) =\(\frac{x}{(x+2)}\) is one-one. Find the inverse of the function f : [-1,1] → Range f.
(Hint: For \(y \in \text { Range } f, y=f(x)=\frac{x}{x+2}\) for some x in [-1,1]
\(x=\frac{2 y}{(1-y)}\)
Answer:

Question 7.
Consider f : R → R given by f(x) = 4x + 3. Show that f is invertible. Find the inverse of f.
Answer:

Question 8.
Consider f : R₊ → [4, ∞) given by f(x) = x² + 4. Show that f is invertible with the
inverse f^-1 of/given by \(f^{-1}(y)=\sqrt{y-4}\) . where R₊ is the set of ail non-negative real numbers.
Answer:

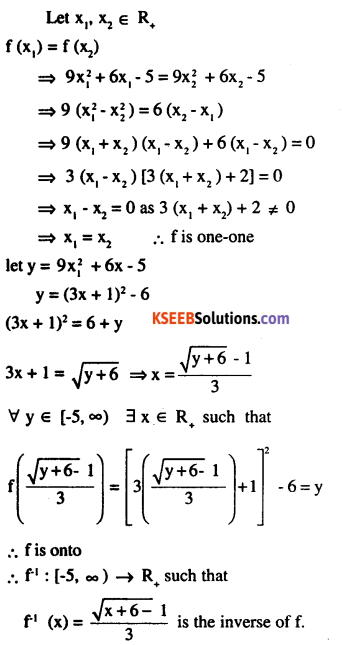
Question 9.
Consider f : R₊ → [- 5, ∞) given by f (x) = 9x³ + 6x – 5. Show that f is invertible with
\(f^{-1}(y)=\left(\frac{(\sqrt{y+6}) \cdot 1}{3}\right)\)
Answer:

 

Question 10.
Let f : X → Y be an invertible function. Show that  f has unique inverse.  (Hint: suppose g₁, and g₂ are two inverses of f. Then for all y ∈ Y, fog₁(y) as 1_Y(y) = fog₂(y). Use one-one ness of A
Answer:
Let g₁ and g₂ are two inverse of f, then for all y ∈ y
fog₁ (y) = f [g (y)] = y = I_y (y)
fog₂ (y) = f [g₂ (y)] = y = I_y (y)
(fog₁) (y) = (fog₂) (y) ∀ y ∈ y
f(g_1,(y)) = f (g₂ (y)) ∀ y ∈ y
∴ g, (y) = g₂ (y) (∴ f is one-one)
g₁ =g₂
⇒ Inverse of f is unique.

Question 11.
Consider f : {1, 2, 3} → {a, b, c} given by f(1) = a,f (2) = b and f(3) = Find f^-1 and show that
(f ^-1)^-1 = f
Answer:
Given that
f = {(1. a), (2, b), (3, c)}
f is one-one and onto
∴ f is invertible f^-1 (a) = 1, f (b) = 2, f^-1 (c) = 3,
∴ f¹ (a, b, c} ⇒ {1,2, 3} is also one and onto
∵ Inverse of f is (f)^-1 exist
(f¹)^-1 = {(1. a), (2, b), (3, c)}=f hence (f^-1)^-1 = f.

Question 12.
Let f : X → Y be an invertible function. Show that the inverse of f^-1 is f, i.e., that (f ^-1)^-1 = f
Answer:
Let f : X → Y is invertible
⇒ f is one and onto and
f^-1 : Y ⇒ X is defined as f^-1(y) = x
y : f (x) ∀ x ∈ X and y ∈ Y
let y₁,y₂ ∈ y
f^-1 (y₁) = f^-2(y₂)
fof¹ (y₁) = fof¹ (y₂)
Iy (y₁) = Iy(y₂)
⇒ y₁ = y₂ ∴ f¹ is one-one
∀ x ∈ X, ∋ y ∈ Y such that
f¹ (y) = x, hence f¹ is onto
hence invertible.
let g = (f¹)^-1
gof^-1 = Iy and f^-1og = lx
∀ x ∈ X, I_x (x) = x
fof^-1(x) = f^-1 [g(x)] =  x
fof^-1 [g (x)] = f (x)
(fof^-1) (g (x)) = f (x)
g (x) = f (x)
g = f
(f^-1)^-1 = f.

Question 13.
If f: R → R be given by \(f(x)=\left(3-x^{3}\right)^{\frac{1}{3}}\),then fof (x) is
(A)\(x^{\frac{1}{3}}\)
(B) x³
(C) x
(D) (3 – x³)
Answer:

Question 14.
Let  \(f: \mathbf{R}-\left\{-\frac{4}{3}\right\} \rightarrow \mathbf{R}\),be a function as \(f(x)=+\frac{4 x}{3 x+4}\).The inverse off is the mag g : Range
\(f \rightarrow \mathbf{R}-\left\{-\frac{4}{3}\right\}\) given by
(A) \(g(y)=\frac{3 y}{3-4 y}\)
(B) \(g(y)=\frac{4 y}{4-3 y}\)
(C) \(g(y)=\frac{4 y}{3-4 y}\)
(D) \(g(y)=\frac{3 y}{4-3 y}\)
Answer: