Students can Download Maths Chapter 3 Axioms, Postulates and Theorems Additional Questions and Answers, Notes Pdf, KSEEB Solutions for Class 8 Maths helps you to revise the complete Karnataka State Board Syllabus and score more marks in your examinations.
Karnataka State Syllabus Class 8 Maths Chapter 3 Axioms, Postulates and Theorems Additional Questions
Question 1.
Choose the correct option
(i) If a = 6 and b = a, then b = 60 by _______
A. Axiom 1
B. Axiom 2
C. Axiom 3
D. Axiom 4
Solution:
A. Axiom 1
(ii) Given a point on the plane, one can draw ________
A. unique
B. two
C. finite numberd
D. infinitely many
Solution:
(D) Infinetly many lines through that point.
(iii) Given two points in a plane, the number of lines which can be drawn to pass through these two points is ________
A. Zero
B. exactly one
C. at most one
D. more than one
Solution:
(B) Exactly one
(iv) If two angles are supplementary, then their sum is
A. 90°
B. 180°
C. 270°
D. 360°
Solution:
(B) 180°
(v) The measure of an angle which is 5 times its supplement is _________
A. 30°
B. 60°
C. 120°
D. 150°
Solution:
(D) 150°
![]()
Question 2.
What is the difference between a pair of supplementary angles and a pair of complementary angles?
Solution:
If the sum of two angles is 180° then they are supplementary angles. If the sum of two angles is 90° then they are complementary angles.
Question 3.
What is the least number of non – collinear points required to determine a plane?
Solution:
Three (3)
Question 4.
When do you say two angles are adjacent?
Solution:
Two angles are said to be adjacent angles if they have a common vertex and a common side.
Question 5.
Let \(\overline{\mathbf{A B}}\) be a segment with C and D between them such that the order of points on the segment is A, C, D, B suppose AD = BC prove that AC = DB
Solution:
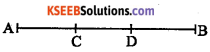
AD = BC [data]
AD – CD = BC – CD [Axiom 3]
AC = DB [In the fig AC – CD = AC , BC – CD = DB]
![]()
Question 6.
Let \(\overrightarrow{\mathbf{A B}}\) and \(\overrightarrow{\mathbf{A B}}\) be two straight lines intersecting at O. Let \(\overrightarrow{\mathbf{O X}}\) be the bisector of ∠BOD. Draw \(\overrightarrow{\mathbf{O Y}}\) between \(\overrightarrow{\mathbf{O D}}\) and \(\overrightarrow{\mathbf{O A}}\) such that \(\overrightarrow{\mathbf{O Y}}\) ⊥ \(\overrightarrow{\mathbf{O B}}\) prove that \(\overrightarrow{\mathbf{O Y}}\) bisects ∠DOA .
Solution:
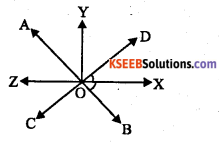
Construction: Produce [/latex] such that \(\overrightarrow{\mathbf{X O}}\) to A point Z such that [/latex] such that \(\overrightarrow{\mathbf{O Z}}\).is between [/latex] such that \(\overrightarrow{\mathbf{O A}}\) and [/latex] such that \(\overrightarrow{\mathbf{O C}}\)
Proof : ∠AOZ = ∠BOX [vertically opposite angles]
∠BOX = ∠POX [\(\overrightarrow{\mathbf{OX}}\) bisects ∠DOB]
∴ ∠AOZ = ∠DOX [Axiom 1] … (i)
[ZOY = [YOX r=90°] … (ii)
(ii) – (i) ∠ZOY – ∠AOZ = ∠YOX – ∠DOX ∠AOY = ∠YOD [Axiom 3]
∴ [/latex] such that \(\overrightarrow{\mathbf{O Y}}\) bisects ∠AOD
Question 7.
Let \(\overrightarrow{\mathbf{A B}}\) and \(\overrightarrow{\mathbf{C D}}\) be two parallel lines and \(\overrightarrow{\mathbf{P Q}}\) be a transversal. Let \(\overrightarrow{\mathbf{P Q}}\) intersect \(\overrightarrow{\mathbf{A B}}\) in L. Suppose the bisector of ∠ALP intersect CD in R and the bisector of ∠PLB intersect CDin S prove that ∠LRS + ∠RSL = 90°
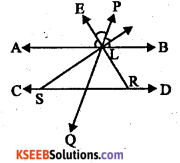
Solution:
∠ALP + ∠BLP = 180° ∠Linear pair]
\(\frac{1}{2}\) ∠ALP + \(\frac{1}{2}\) ∠BLP = \(\frac{1}{2}\)180°
[Multiplying by \(\frac{1}{2}\) ]
∠ELP + ∠FLP = 90° [∴ \(\overrightarrow{\mathbf{E L}}\) and \(\overrightarrow{\mathbf{F L}}\) are bisectors of ∠ALP and ∠BLP ]
∠ELF = 90°
[SLR = ∠ELF = 90°
[vertically opposite angles]
In ∆ SLR, ∠SLR + ∠LRS + ∠RSL = 180° [sum of the angles of the triangle is 180° ]
90 + ∠LRS + ∠RSL = 180°
∠LRS + ∠SL = 180 – 90
∠LRS + [RSL = 90°
![]()
Question 8.
In the adjoining figure \(\overrightarrow{\mathrm{AB}}\) and \(\overrightarrow{\mathrm{CD}}\) are parallel lines. The transversals \(\overrightarrow{\mathrm{PQ}}\) and \(\overrightarrow{\mathrm{RS}}\) intersect at U on the line \(\overrightarrow{\mathrm{AB}}\). Given ∠DWU = 110° and ∠CVP = 70° find the measure of ∠QUS.
Solution:
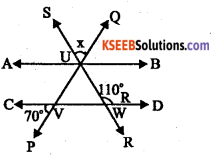
∠ AUV – ∠CVP = 70°
[corresponding angles]
∠QUB – ∠AUV = 70° [vertically opposite angles]
∠BUW + ∠DWU = 180° [Interior angles on the same side of transversal]
∠BUW + 110°= 180°
∠BUW = 180-110° = 70°
∠BUW = ∠AUS= 70° [vertically opposite angles]
∠AUS + ∠SUQ + ∠QUB = 180° ∠AUB is a straight angle]
70 + ∠SUQ + 70 = 180°
∠SUQ + 140° = 180°
∠SUQ = 180° – 140°
∠SUQ = 40°
![]()
Question 9.
What is the angle between the hours hand and minutes hand of a clock at (i) 1.40 hours (ii) 2.15 hours [use 1° = 60 minutes]
Solution:
i. 1.40 hours – 190°
ii. 2.15 hours – 22° 30′
Question 10.
How much would hour’s hand have moved from its position at 12 noon when the time is 4.24 p.m?
Solution:
144°
Question 11.
Let \(\overrightarrow{\mathrm{AB}}\) be a line segment and let C be the midpoint of \(\overrightarrow{\mathrm{AB}}\) Extend AB to D such that B lies between A and D. Prove that AD + BD = 2CD
Solution:
AD + BD = 2CD L.H.S – AD + BD
= AC + CB + BD + BD [∵ AD = AC + CB + BD]
= CB + CB + BD + BD
[∴ AC = CB]
= CB + BD + CB + BD
= CD + CD
= 2CD = RHS
∴ AD + B D = 2CD
![]()
Question 12.
Let \(\overrightarrow{\mathbf{A B}}\) and \(\overrightarrow{\mathbf{C D}}\) be two lines . intersecting at a point O. Let \(\overrightarrow{\mathbf{O X}}\) be a ray bisecting [BOD. Prove that the extension of \(\overrightarrow{\mathbf{O X}}\) to the left of O bisects ∠AOC.
Solution:

∠DOX = ∠BOX [ ∵\(\overrightarrow{\mathbf{O X}}\) bisects ∠BOD]
∠DOX =∠COY [vertically opposite angles] ….(i)
∠BOX = ∠AOY
[vertically opposite anglesj … (ii)
From (i) and (ii)
[vertically opposite] …(ii)
∠AQY – ∠COY [Axiom 1]
∴ \(\overrightarrow{\mathbf{O X}}\) bisects ∠AOC
Question 13.
Let \(\overrightarrow{\mathbf{O X}}\) be a ray and let \(\overrightarrow{\mathbf{O A}}\) and \(\overrightarrow{\mathbf{O B}}\) be two rays on the same side of \(\overrightarrow{\mathbf{O X}}\) with \(\overrightarrow{\mathbf{O A}}\) between \(\overrightarrow{\mathbf{O X}}\) and \(\overrightarrow{\mathbf{O B}}\) . Let \(\overrightarrow{\mathbf{O C}}\) be the bisector of ∠AOB prove that ∠XOA + ∠XOB = 2∠XOC
Solution:
∠XOA + ∠XOB = 2∠XOC
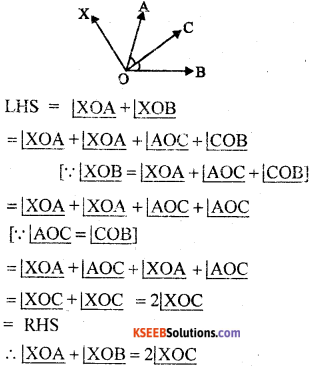
![]()
Question 14.
Let \(\overrightarrow{\mathbf{O A}}\) and \(\overrightarrow{\mathbf{O B}}\) be two rays and let \(\overrightarrow{\mathbf{O X}}\) be a ray between \(\overrightarrow{\mathbf{O A}}\) and \(\overrightarrow{\mathbf{O B}}\) such ∠AOX > ∠XOB . Let \(\overrightarrow{\mathbf{O C}}\) be the bisector of ∠AOB prove that ∠AOX – ∠XQB = 2∠COX
Solution:
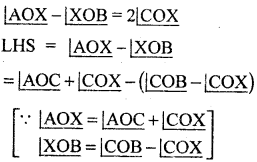
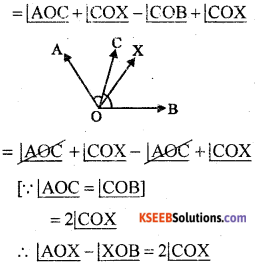
Question 15.
Let \(\overrightarrow{\mathbf{OA}}\), \(\overrightarrow{\mathbf{O B}}\), \(\overrightarrow{\mathbf{OC}}\) be three rays such that \(\overrightarrow{\mathbf{OC}}\) lies between \(\overrightarrow{\mathbf{OA}}\) and \(\overrightarrow{\mathbf{O A}}\). Suppose the bisectors of ∠AOC and ∠COB are perpendicular to each other. Prove that B, O. A are . collinear.
Solution:
∠XOC + ∠YOC – 90° [data]
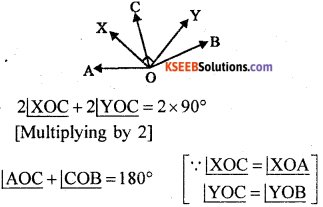
∠AOB = 180°
A, O and B are collinear.
![]()
Question 16.
In the adjoining figure \(\overrightarrow{\mathrm{AB}}\) || \(\overrightarrow{\mathrm{DE}}\) . Prove that ∠ABC – [DCB + [CDE = 180°
Solution:
Construction: Through C draw \(\overrightarrow{\mathrm{XY}}\) || \(\overrightarrow{\mathrm{AB}}\)
Proof : ∠ABC + ∠BCX = 180° [sum of interior angles on the same side of trnasversal]
∴ ∠BCX = 180 – ∠ABC
∠CDE + ∠DCY – 180° [sum of interior angles on the same side of transversal]
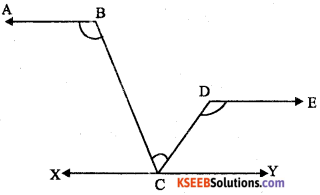
∴ ∠DCY = 180° – ∠CDE
∠BCX + ∠BCD + ∠DCY = 180° [∵ XCY is straight angle]
180 – ∠ABC + ∠BCD + 180 – ∠CDE = 180° [By substituting]
360 – ∠ABC + ∠BCD – ∠CDE – 180°
360 – 180 – ∠ABC – ∠BCD + CDE
180° = ∠ABC – ∠BCD + ∠CDE
or
∠ABC – ∠BCD + ∠CDE = 180°
Question 17.
Consider two parallel lines and a transversal among the measures of 8 angles formed how many distinct numbers are there?
Solution:
There will be two distinct numbers.
![]()