KSEEB Solutions for Class 7 Maths Chapter 13 Exponents and Powers Ex 13.2

Students can Download Chapter 13 Exponents and Powers Ex 13.2, Question and Answers, Notes Pdf, KSEEB Solutions for Class 7 Maths, Karnataka State Board Solutions help you to revise complete Syllabus and score more marks in your examinations.

Karnataka State Syllabus Class 7 Maths Chapter 13 Exponents and Powers Ex 13.2

Question 1.
Using laws of exponents, simplify and write the answer in exponential form :
(i) 3² × 3⁴ × 3⁸
(ii) 6¹⁵ ÷ 6¹⁰
(iii) a³ × a²
(iv) 7^x × 7²
(v) (5²)³ ÷ 5²
(vi) 2⁵ × 5⁵
(vii) (a⁴ × b⁴)
(viii) (3⁴)³
(ix) (2²⁰ ÷ 2¹⁵) × 2³
(x) 8^t ÷ 8²
Solution:
i) 3² × 3⁴ × 3⁸
= 3^{2 + 4 + 8} = 3¹⁴ [∵ a^m × aⁿ = a^{m + n}]

ii) 6¹⁵ ÷ 6¹⁰
= 6^{15 – 10} = 6⁵ [∵ a^m ÷ aⁿ = a^{m – n}]

iii) a³ × a²
= a^{3 + 2} = a⁵ [∵ a^m × aⁿ = a^{m + n}]

iv) 7^x × 7²
= 7^{x + 2} [∵ a^m × aⁿ = a^{m + n}]

v) (5²)³ ÷ 5³
= 5^{2 × 3} ÷ 5³ = 5^{6 – 3} [(a^m)ⁿ = a^mn
∵ a^m ÷ aⁿ = a^{m – n}]

vi) 2⁵ × 5⁵
= (2 × 5)⁵ = 10⁵ [∵ a^m × b^m = (ab)^m]

vii) a⁴ × b⁴
= a⁴ × b⁴ = (ab)⁴ [∵ a^m × b^m = (ab)^m]

viii) (3⁴)³
= 3^{4 × 3} = 3¹² [(a^m)ⁿ = a^mn]

ix) (2²⁰ ÷ 2¹⁵)
(2²⁰ ÷ 2¹⁵) × 2³ = 2^{20 – 15} × 2³ [a^m ÷ aⁿ = a^{m – n}]
= 2⁵ × 2³
= 2^{5 + 3} = 2⁸ [a^m × aⁿ = a^{m + n}]

x) 8^t ÷ 8²
= 8^{t – 2} [∵ a^m ÷ aⁿ = a^{m – n}]

Question 2.
Simplify and express each of the following in exponential form :
i)

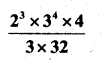
Solution:

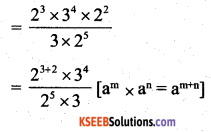
ii) ((5²)³ × 5⁴) ÷ 5⁷
= (5^{2 × 3} × 5⁴) ÷ 5⁷ ((a^m)ⁿ = a^mn)
= 5⁶ × 5⁴ ÷ 5⁷
= 5^{6 + 4} ÷ 5⁷ = 5^{10 – 7} = 5³

iii) 25⁴ ÷ 5³
= (5²)⁴ ÷ 5³ = 5^{2 × 4} ÷ 5³
= 5⁸ ÷ 5³ = 5^{8 – 3} = 5⁵ [a^m ÷ aⁿ = a^{m – n}

iv)


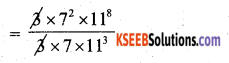
= 7^{2 – 1} × 11^{8 – 3} [a^m ÷ aⁿ = a^{m – n}]
= 7¹ × 11⁵ = 7 × 11⁵

vi) 2⁰ + 3⁰ + 4⁰
= 1 + 1 + 1 = [3] [a⁰ = 1]

vii) 2⁰ × 3⁰ × 4⁰
= 1 × 1 × 1 = 1

viii) (3⁰ + 2⁰) × 5⁰
= (1 + 1) × 1 = 2 × 1 = 2

ix)

= (2a)²

x)

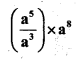
= a ^{5 – 3} × a⁸
= a² × a⁸ a^{2 + 8}

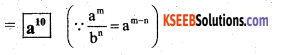
xi)

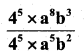
xii) (2³ × 2)²
= (2³ × 2¹)²
= (2⁴)²
= 2^{4 × 2} = 2⁸

Question 3.
Say true or false and justify your answer:
i) 10 × 10¹¹ = 100¹¹
L.H.S = 10 × 10¹¹ = 10^{1 + 11} = 10¹² RHS
100¹¹ = (10 × 10)¹¹
= (10²)¹¹
= 1o² × 11 = 10²²
∴ 10¹² ≠ 10²²
So 10 × 10¹¹ ≠ 100¹¹ ∴ It is false.

ii) 2³ > 5²
= 2 × 2 × 2 > 5 × 5
= 8 > 25
∴ 2³ > 5² ∴ It is false.

iii) 2³ × 3² = 6⁵
= 2 × 2 × 2 × 3 × 3
= 6 × 6 × 6 × 6 × 6
= 72 = 7776
∴ 2³ × 3² ≠ 6⁵ ∴ It is false.

iv) 3⁰ = (1000)⁰
1 = 1
∴ 3⁰ = (1000)⁰ ∴ It is true

Question 4.
Express each of the following as a product of prime factors only in exponential form :

i) 108 x 192

= 2 × 2 × 3 × 3 × 3 2 × 2 × 2 × 2 × 2 × 2 × 3
= 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3
= 2⁸ × 3⁴

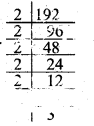
It is the product of prime factor

ii) 270
270 = 2 × 3 × 3 × 3 × 5
= 2 × 3³ × 5

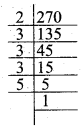
It is required prime factor.

iii) 729 × 64
729 = 3 × 3 × 3 × 3 × 3 × 3
64 = 2 × 2 × 2 × 2 × 2 × 2
∴ 729 × 64 = 3⁶ × 2⁶

It is the required prime factor.

iv) 768
768 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3
= 2⁸ × 3¹ or = 2⁸ × 3

It is a required prime factor.

Question 5.
Simplify :
i)

Solution:

ii)

Solution:

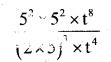
iii)

Solution: